This course is the second course in the Linear Algebra Specialization. In this course, we continue to develop the techniques and theory to study matrices as special linear transformations (functions) on vectors. In particular, we develop techniques to manipulate matrices algebraically. This will allow us to better analyze and solve systems of linear equations. Furthermore, the definitions and theorems presented in the course allow use to identify the properties of an invertible matrix, identify relevant subspaces in R^n,



Linear Algebra: Matrix Algebra, Determinants, & Eigenvectors
Dieser Kurs ist Teil von Spezialisierung Linear Algebra from Elementary to Advanced

Dozent: Joseph W. Cutrone, PhD
TOP-LEHRKRAFT
3.836 bereits angemeldet
Bei  enthalten
enthalten
(52 Bewertungen)
(52 Bewertungen)
Wichtige Details

Zu Ihrem LinkedIn-Profil hinzufügen
18 Aufgaben
Erfahren Sie, wie Mitarbeiter führender Unternehmen gefragte Kompetenzen erwerben.

Erweitern Sie Ihre Fachkenntnisse
- Lernen Sie neue Konzepte von Branchenexperten
- Gewinnen Sie ein Grundverständnis bestimmter Themen oder Tools
- Erwerben Sie berufsrelevante Kompetenzen durch praktische Projekte
- Erwerben Sie ein Berufszertifikat zur Vorlage


Erwerben Sie ein Karrierezertifikat.
Fügen Sie diese Qualifikation zur Ihrem LinkedIn-Profil oder Ihrem Lebenslauf hinzu.
Teilen Sie es in den sozialen Medien und in Ihrer Leistungsbeurteilung.

In diesem Kurs gibt es 6 Module
In this module, we now look at what arithmetic operation we can perform on nxm matrices and how these operations correspond to operations on functions. In particular, we will view at matrix multiplication AB as a composition of function A(B(x)). In this way, algebraic properties like non-commutativity will become more apparent. We will also look for those matrices that are invertible. Since we no longer have the Horizontal Line Test, new tests for invertibility will be needed. This will lead to the study of the very important matrix invariant, the determinant.
Das ist alles enthalten
3 Videos2 Lektüren3 Aufgaben
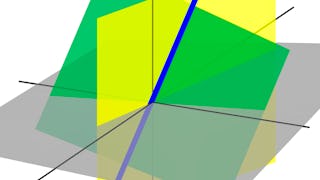
In this module we investigate the structure of R^n by formally defining the notion of a subspace. These special sets are those that look like smaller versions of R^n that pass through the origin. These subsets have invariants called a dimension which captures a notion of size. The linear algebra definition of dimension, which uses the notion of linearly independent vectors, matches our intuition in low dimensions where lines have dimension one and planes have dimension two. These sets, and their sizes, turn out to be another tool to student matrices as functions as both the zeros and image of a matrix are subspaces of R^n.
Das ist alles enthalten
2 Videos2 Lektüren3 Aufgaben
The determinant is a real number calculated from a square matrix that determines the invertibility of a square matrix. Its value characterizes the invertibility of the matrix. The determinant also has a geometric meaning: the absolute value of the determinant scales the volumes of sets under the function. In this module, we will show how to calculate the determinant of nxn matrices and study its properties.
Das ist alles enthalten
3 Videos3 Lektüren4 Aufgaben
In this module we study special vectors, called eigenvectors, of a linear transformation defined by a square matrix A. These are vectors whose image is easily visualized as they are scaled by a real number called the eigenvalue. While eigenvalues can be complex numbers, we do not consider that case in this course. Eigenvalues and eigenvectors are central to the theory of discrete dynamical systems, differential equations, and Markov chains and the eigentheory presented here also appear in settings in more advanced pure math courses.
Das ist alles enthalten
2 Videos2 Lektüren3 Aufgaben
In this module we continue our study of eigenvalues and eigenvectors, in particular how they relate to diagonalizable matrices. Eigenvectors are so important: they make understanding linear transformations easy. They are the "axes" (directions) along which a linear transformation acts simply by "stretching/compressing" and/or "flipping"; eigenvalues give you the factors by which this compression occurs. The more directions you have along which you understand the behavior of a linear transformation, the easier it is to understand the linear transformation; so you want to have as many linearly independent eigenvectors as possible associated to a single linear transformation.
Das ist alles enthalten
2 Videos2 Lektüren3 Aufgaben
Congratulations on reaching the final assessment! Review all vocabulary and theorems before attempting the final quiz below. Think about what each theorem is saying both algebraically as well as geometrically. Provide examples (with pictures in R^2 and R^3) along with counterexamples of each theorem and vocabulary term. Lastly, be sure to work through some examples for computation, looking for any of the shortcuts in the calculations when possible. In addition, there is an optional project that applies the theory of this course. You will see how eigenvalues and eigenvectors are applied to Markov Chains and the Google Page Rank algorithm. I strongly recommend you attempt this project. Good luck!
Das ist alles enthalten
1 Lektüre2 Aufgaben
Dozent

Empfohlen, wenn Sie sich für Machine Learning interessieren

Johns Hopkins University

Johns Hopkins University

The University of Sydney


IIT Roorkee
Warum entscheiden sich Menschen für Coursera für ihre Karriere?




Bewertungen von Lernenden
52 Bewertungen
- 5 stars
86,53 %
- 4 stars
11,53 %
- 3 stars
1,92 %
- 2 stars
0 %
- 1 star
0 %
Zeigt 3 von 52 an
Geprüft am 31. Okt. 2024
Good course content. Instructor is inspiring, quite able to view thing from different perspective and useful to increase understanding of concept.
Geprüft am 6. Juni 2024
Great course! Really explains every topic clearly. Go watch the 3Blue1Brown videos, they're the best
Geprüft am 27. Dez. 2023
It helps me to advance my knowledge and the way of teaching is just to the point, that's what i was looking for.
I would say it would be great if their are more examples included.

Neue Karrieremöglichkeiten mit Coursera Plus
Unbegrenzter Zugang zu 10,000+ Weltklasse-Kursen, praktischen Projekten und berufsqualifizierenden Zertifikatsprogrammen - alles in Ihrem Abonnement enthalten
Bringen Sie Ihre Karriere mit einem Online-Abschluss voran.
Erwerben Sie einen Abschluss von erstklassigen Universitäten – 100 % online
Schließen Sie sich mehr als 3.400 Unternehmen in aller Welt an, die sich für Coursera for Business entschieden haben.
Schulen Sie Ihre Mitarbeiter*innen, um sich in der digitalen Wirtschaft zu behaupten.
Häufig gestellte Fragen
Access to lectures and assignments depends on your type of enrollment. If you take a course in audit mode, you will be able to see most course materials for free. To access graded assignments and to earn a Certificate, you will need to purchase the Certificate experience, during or after your audit. If you don't see the audit option:
The course may not offer an audit option. You can try a Free Trial instead, or apply for Financial Aid.
The course may offer 'Full Course, No Certificate' instead. This option lets you see all course materials, submit required assessments, and get a final grade. This also means that you will not be able to purchase a Certificate experience.
When you enroll in the course, you get access to all of the courses in the Specialization, and you earn a certificate when you complete the work. Your electronic Certificate will be added to your Accomplishments page - from there, you can print your Certificate or add it to your LinkedIn profile. If you only want to read and view the course content, you can audit the course for free.
If you subscribed, you get a 7-day free trial during which you can cancel at no penalty. After that, we don’t give refunds, but you can cancel your subscription at any time. See our full refund policy.

